How to Create a Monthly Accrued Interest Calculator in Excel – 4 Methods

Monthly Accrued Interest Calculator in Excel

The Accrued Interest is the payable or receivable interest on a loan or bond after a period of time.

Download the Excel Template

Download the Template. Monthly Accrued Interest Calculator.xlsx

Accrued Interest

The formula to calculate the accrued interest is: Accrued Interest = Loan Amount or Par Value ✖ ( Yearly Interest/365)✖ Period of Interest Accrued

Method 1 – Applying the Accrued Interest Formula

Provide Loan Amount, Annual Interest Rate, and Accrued Interest Period to find the accrued interest amount.

Accrued Interest = Loan Amount or Par Value ✖ ( Yearly Interest/365)✖ Period of Interest Accrued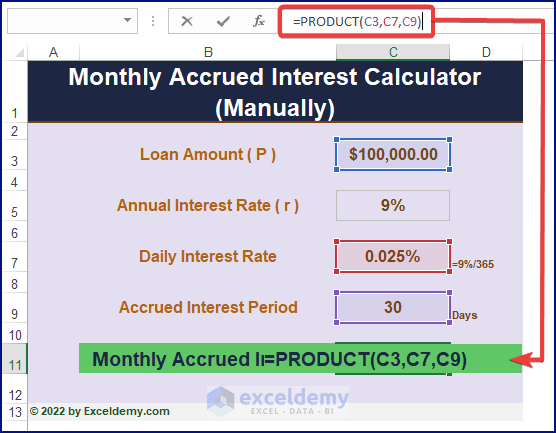
=PRODUCT(C3,C7,C9)

Accrued Interest Formula

Formula Breakdown

Method 2 – Using the ACCRINT Function

=ACCRINT(issue, first_interest, settlement, rate, par, frequency, [basis], [calc_method])

The Arguments

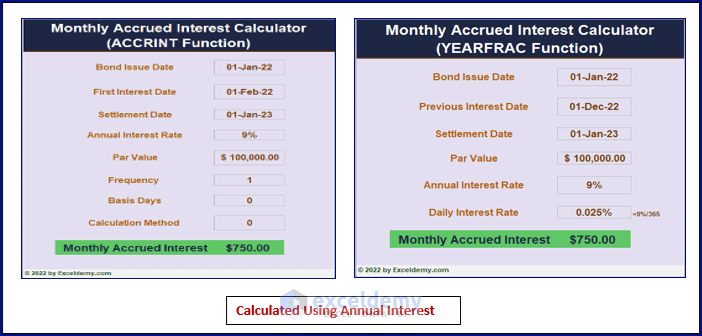
Issue: The date when a loan or bond is issued.

First_interest: The date of the first interest payment.

Settlement: The end date of the loan.

Rate: Annual or Yearly Interest rate.

Par: The loan amount.

Frequency: The annual number of loan payments. 1 for Annual, 2 for Semi-annual, and 4 for Quarterly payments.

Basis: The basis is set to 0 if the argument is omitted. [Optional]

Calculation_method: It’s either 0 or 1 (calculates accrued interest from the First_interest date to the Settlement date). [Optional]

=ACCRINT(C3,C5,C7,C9,C11,C13,C15,C17)/12

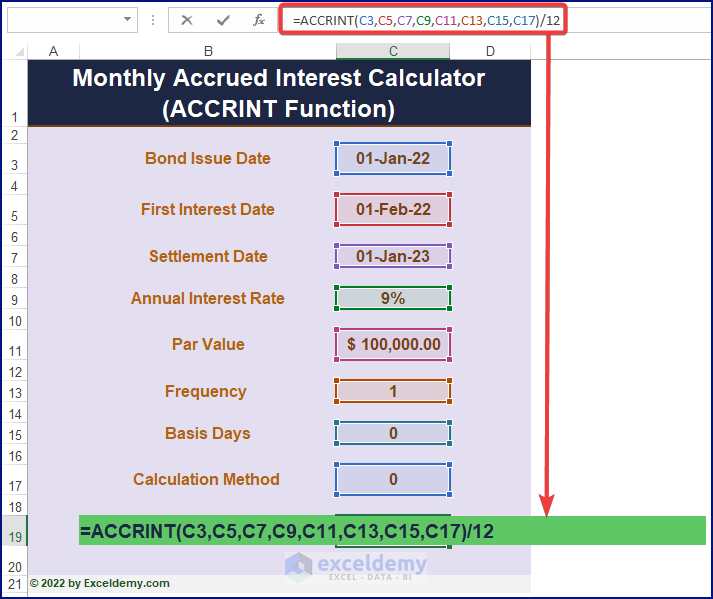
ACCRINT Function to be used as Monthly Accrued Interest Calculator in Excel

Method 3 – Counting Days Using the DAYS360 Function

Days360(start_date,end_date,[method])

Multiplying the outcome by the Daily Interest Rate and Par Value will return the monthly accrued interest. Make sure the difference between the two dates is one (1) month.

=DAYS360(C5,C7,FALSE)*C9*C13

Method 4 – Finding the Year Fraction using the YEARFRAC Function

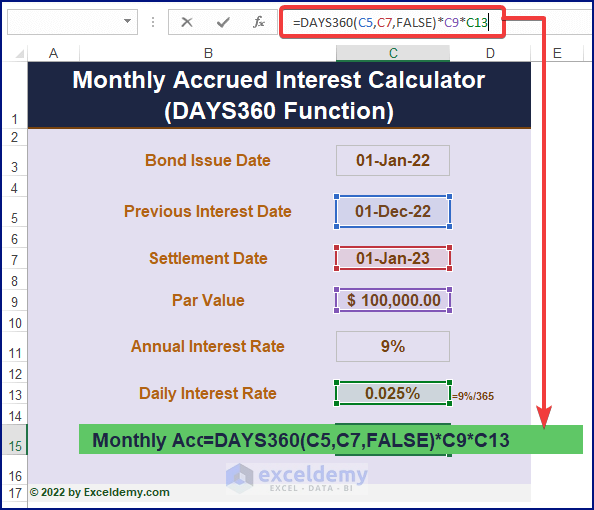
The syntax of the function is:

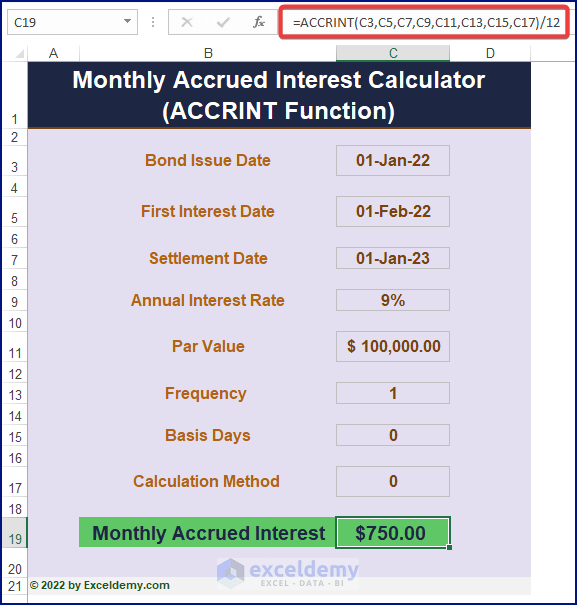
YearFrac(start_date, end_date, [basis])

The returned value is multiplied by 365, Par Value, and Annual Rate to display the accrued interest. For the monthly accrued interest, the two dates must be one month apart.

=YEARFRAC(C5,C7,0)*365*C9*C13

Cross-checking the Accrued Interest Value

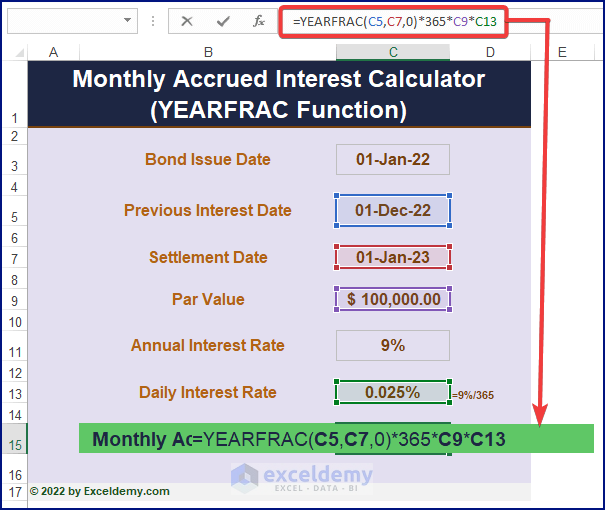
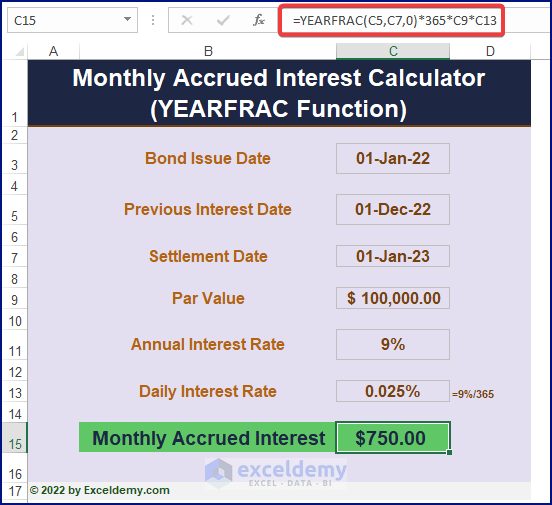
Two different values are returned for the monthly accrued interest because two different approaches were used:

a. Daily Interest Rate

It takes the annual interest rate and divides it by 365. This result is multiplied by the Par Value, Daily Interest Rate and 30 (days in a month).

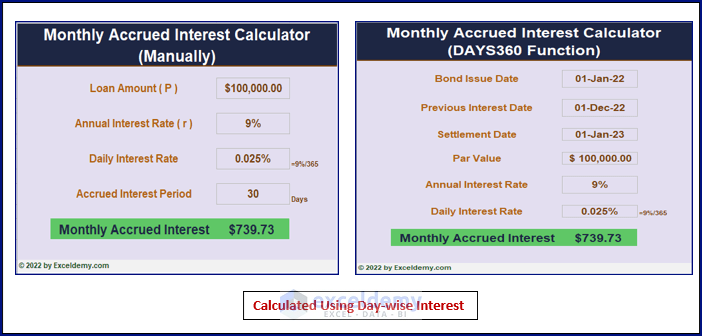
Monthly Accrued Interest Calculator in Excel-Daily Interest

b. Annual Interest Rate

The ACCRINT formula calculates the annual accrued interest and, by dividing it by 12, returns the monthly accrued interest.

Monthly Accrued Interest Calculator in Excel-Annual Interest

Related Articles